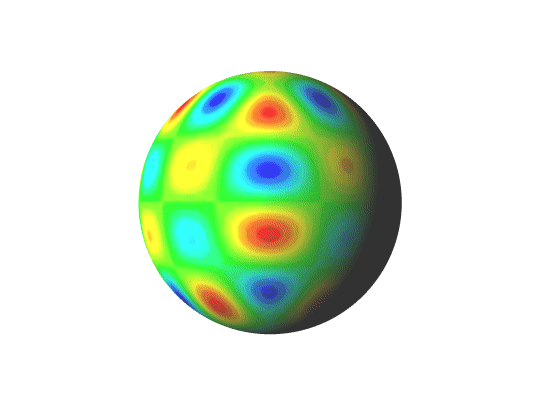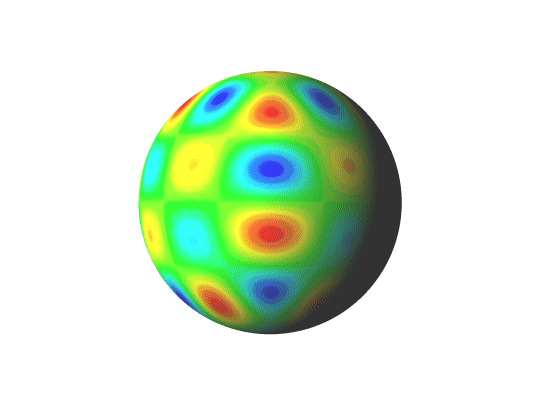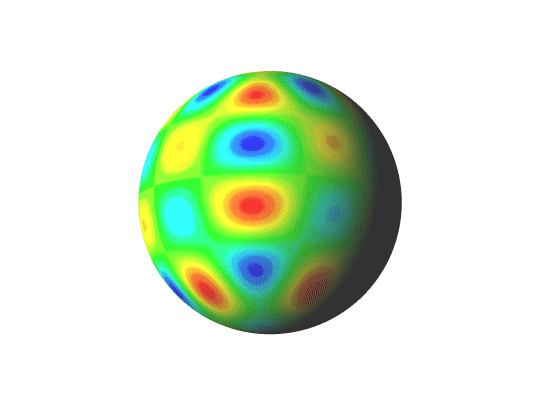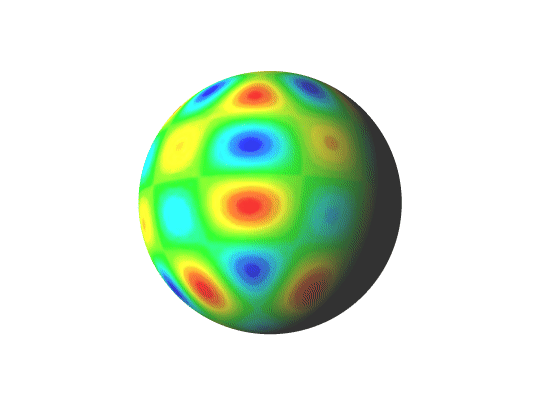
正四面体型調和関数(その2)
\(L=8\)の基底を取り出す
\(2\)つの\(L=4\)の合成系は、
\begin{align*}
|\psi_{4}>|\psi_{4}>&=\frac{1}{24}(\sqrt{5}(|4,4>+|4,-4>)+\sqrt{2\cdot 7}|4,0>)(\sqrt{5}(|4,4>+|4,-4>)+\sqrt{2\cdot 7}|4,0>) \\
&=\frac{1}{24}(5(|4,4,4,4>+|4,4,4,-4>+|4,4,-4,4>+|4,4,-4,-4>) \\
&\ +\sqrt{2\cdot 5\cdot 7}(|4,4,4,0>+|4,4,-4,0>+|4,4,0,4>+|4,4,0,-4>)+\sqrt{2\cdot 7}|4,4,0,0>)
\end{align*}
であるが、そのうちの\(L=8\)の成分に注目すると、\(3j\)記号を用いて、
\begin{align*}
<8,8|4,4,4,4>=(-1)^{4-4+8}\sqrt{2\cdot 8+1}\begin{pmatrix} 4 & 4 & 8 \\ 4 & 4 & -8 \end{pmatrix},\
& \begin{pmatrix} 4 & 4 & 8 \\ 4 & 4 & -8 \end{pmatrix} =\sqrt{\frac{1}{17}} \\
<8,4|4,4,4,0>=(-1)^{4-4+8}\sqrt{2\cdot 8+1}\begin{pmatrix} 4 & 4 & 4 \\ 4 & 0 & -4 \end{pmatrix},\
& \begin{pmatrix} 4 & 4 & 4 \\ 4 & 0 & -4 \end{pmatrix} =\sqrt{\frac{1}{2\cdot 3\cdot 17}} \\
<8,0|4,4,4,-4>=(-1)^{4-4+8}\sqrt{2\cdot 8+1}\begin{pmatrix} 4 & 4 & 0 \\ 4 & -4 & 0 \end{pmatrix},\
& \begin{pmatrix} 4 & 4 & 0 \\ 4 & -4 & 0 \end{pmatrix} =\frac{1}{3}\sqrt{\frac{1}{2\cdot 5\cdot 11\cdot 13\cdot 17}} \\
<8,0|4,4,0,0>=(-1)^{4-4+8}\sqrt{2\cdot 8+1}\begin{pmatrix} 4 & 4 & 0 \\ 0 & 0 & 0 \end{pmatrix},\
& \begin{pmatrix} 4 & 4 & 0 \\ 0 & 0 & 0 \end{pmatrix} =\frac{5}{3}\sqrt{\frac{2\cdot 5}{11\cdot 13\cdot 17}} \\
\end{align*}
\[<8,-4|4,4,-4,0>=(-1)^{4+4-8}<8,4|4,4,4,0>=<8,4|4,4,4,0>\]
\[<8,-8|4,4,-4,-4>=(-1)^{4+4-8}<8,8|4,4,4,4>=<8,8|4,4,4,4>\]
より、
\[|\psi_{8}>\propto 5\sqrt{\frac{1}{17}}(|8,8>+|8,-8>)+2\sqrt{\frac{5\cdot 7}{13\cdot 17}}(|8,4>+|8,-4>)+3\sqrt{\frac{2\cdot 5\cdot 11}{13\cdot 17}}|8,0>\]
なので、規格化条件から、
\[|\psi_{8}>=\frac{1}{48}\sqrt{2\cdot 3\cdot 5\cdot 13}(|8,8>+|8,-8>)+\frac{1}{24}\sqrt{2\cdot 3\cdot 7}(|8,4>+|8,-4>)+\frac{1}{8}\sqrt{3\cdot 11}|8,0>\]
である。この基底は\(z\)軸回りの\(1/4\)回転について不変で、正八面体の対称性ももつ
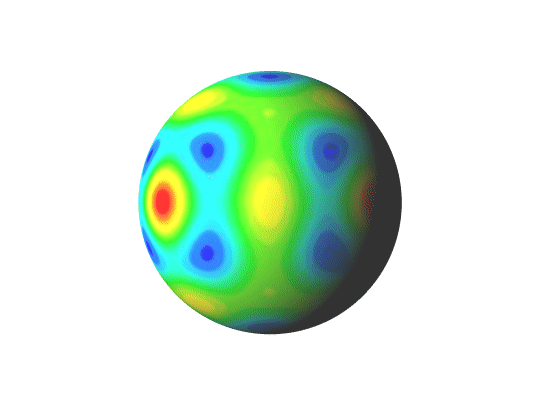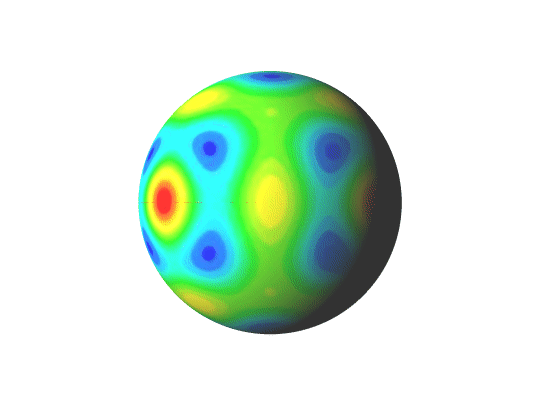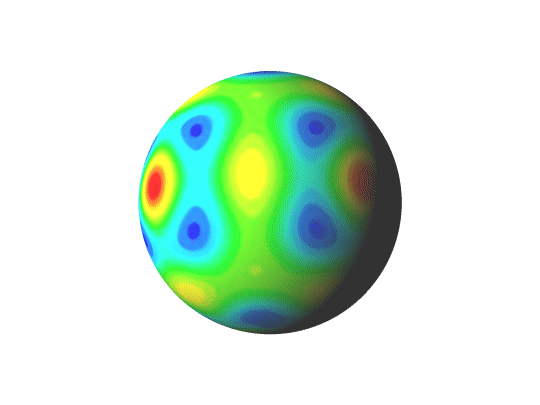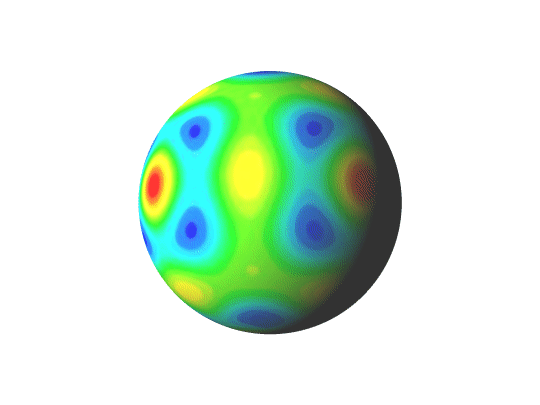
\(L=9\)の基底を取り出す
\(L=9\)の基底は、\(A_{1}\)表現の基底と\(A_{2}\)表現の基底が1つずつある。\(A_{1}\)表現の基底は、\(|\psi_{3}>\)と\(|\psi_{6,o}>\)の合成系から取り出す。
\begin{align*}
|\psi_{3}>|\psi_{6,o}>&=\frac{1}{4\sqrt{2}}(|3,2> - |3,-2>)(\sqrt{7}(|6,4>+|6,-4>)-\sqrt{2}|6,0>) \\
&=\frac{1}{4\sqrt{2}}(\sqrt{7}(|3,6,2,4>+|3,6,2,-4>-|3,6,-2,4>-|3,6,-2,-4>) \\
&-\sqrt{2}(|3,6,2,0>-|3,6,-2,0>))
\end{align*}
であるが、そのうちの\(L=9\)の成分に注目すると、\(3j\)記号を用いて、
\begin{align*}
<9,6|3,6,2,4>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ 2 & 4 & -6 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ 2 & 4 & -6 \end{pmatrix} =-\frac{1}{2}\sqrt{\frac{3\cdot 11}{17\cdot 19}} \\
<9,2|3,6,-2,4>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ -2 & 4 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ -2 & 4 & -2 \end{pmatrix} =-\frac{1}{2}\sqrt{\frac{11}{13\cdot 17\cdot 19}} \\
<9,2|3,6,2,0>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ 2 & 0 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ 2 & 0 & -2 \end{pmatrix} =-\sqrt{\frac{7\cdot 11}{2\cdot 13\cdot 17\cdot 19}} \\
\end{align*}
\[<9,-2|3,6,-2,0>=(-1)^{3+6-9}<9,2|3,6,2,0>=<9,2|3,6,2,0>\]
\[<9,-2|3,6,2,-4>=(-1)^{3+6-9}<9,2|3,6,-2,4>=<9,2|3,6,-2,4>\]
\[<9,-6|3,6,-2,-4>=(-1)^{3+6-9}<9,6|3,6,2,4>=<9,6|3,6,2,4>\]
より、
\[|\psi_{9}>\propto -\frac{1}{2}\sqrt{\frac{3\cdot 7\cdot 11}{17\cdot 19}}(|9,6>-|9,-6>)+\frac{3}{2}\sqrt{\frac{7\cdot 11}{13\cdot 17\cdot 19}}(|9,2>-|9,-2>)\]
なので、規格化条件から、
\[|\psi_{9}>=-\frac{1}{8}\sqrt{2\cdot 13}(|9,6>-|9,-6>)+\frac{1}{8}\sqrt{2\cdot 3}(|9,2>-|9,-2>)\]
である。
\(A_{2}\)表現の基底は、\(|\psi_{3}>\)と\(|\psi_{6,o}>\)の合成系から取り出す。
\begin{align*}
|\psi_{3}>|\psi_{6,t}>&=\frac{1}{8}(|3,2> - |3,-2>)(\sqrt{5}(|6,6>+|6,-6>)-\sqrt{11}(|6,2>+|6,-2>)) \\
&=\frac{1}{8}(\sqrt{5}(|3,6,2,6>+|3,6,2,6>-|3,6,-2,6>-|3,6,-2,-6>) \\
&-\sqrt{11}(|3,6,2,2>+|3,6,2,-2>-|3,6,-2,2>-|3,6,-2,-2>))
\end{align*}
であるが、そのうちの\(L=9\)の成分に注目すると、\(3j\)記号を用いて、
\begin{align*}
<9,8|3,6,2,6>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ 2 & 6 & -8 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ 2 & 6 & -8 \end{pmatrix} =-\sqrt{\frac{1}{3\cdot 19}} \\
<9,4|3,6,-2,6>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ -2 & 6 & -4 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ -2 & 6 & -4 \end{pmatrix} =-\frac{1}{2}\sqrt{\frac{1}{3\cdot 7\cdot 17\cdot 19}} \\
<9,4|3,6,2,2>=(-1)^{3-6+9}\sqrt{2\cdot 9+1}\begin{pmatrix} 3 & 6 & 9 \\ 2 & 2 & -4 \end{pmatrix},\
& \begin{pmatrix} 3 & 6 & 9 \\ 2 & 2 & -4 \end{pmatrix} =-\frac{1}{2}\sqrt{\frac{3\cdot 5\cdot 11}{3\cdot 7\cdot 17\cdot 19}} \\
\end{align*}
\[<9,-4|3,6,-2,-2>=(-1)^{3+6-9}<9,4|3,6,2,2>=<9,4|3,6,2,2>\]
\[<9,-4|3,6,2,-6>=(-1)^{3+6-9}<9,4|3,6,-2,6>=<9,4|3,6,-2,6>\]
\[<9,-8|3,6,-2,-6>=(-1)^{3+6-9}<9,8|3,6,2,6>=<9,8|3,6,2,6>\]
より、
\[|\psi_{9}>\propto -\sqrt{\frac{5}{3\cdot 19}}(|9,8>-|9,-8>)+\sqrt{\frac{5\cdot 17}{3\cdot 17\cdot 19}}(|9,4>-|9,-4>)\]
なので、規格化条件から、
\[|\psi_{9}>=-\frac{1}{12}\sqrt{3\cdot 7}(|9,8>-|9,-8>)+\frac{1}{12}\sqrt{3\cdot 17}(|9,4>-|9,-4>)\]
である。
\(L=9\)の\(A_{2}\)表現の基底は\(z\)軸回りの\(1/4\)回転について不変で、正八面体の対称性ももつので、
\[|\psi_{9,o}>=-\frac{1}{12}\sqrt{3\cdot 7}(|9,8>-|9,-8>)+\frac{1}{12}\sqrt{3\cdot 17}(|9,4>-|9,-4>)\]
と表し、\(A_{1}\)表現の基底は、
\[|\psi_{9,t}>=-\frac{1}{8}\sqrt{2\cdot 13}(|9,6>-|9,-6>)+\frac{1}{8}\sqrt{2\cdot 3}(|9,2>-|9,-2>)\]
と表して区別する。